The multiple images of an object that is located between two (almost) parallel mirrors illustrate the principle of creating superradiant emission of x-rays. If an ensemble of atoms between two mirrors is resonantly excited by x-rays of a synchrotron, its multiple images add up and emit a fast, superradiant radiation pulse that exhibits a redshift of its frequency, the collective Lamb shift. (Photo: Deutsches Elektronen-Synchrotron DESY, Hamburg, Boris Kumicak)
Cooperative emission describes a number of optical phenomena that result from the collective interaction of an ensemble of identical two-level atoms with a common, resonant radiation field. The most prominent phenomenon in this field is superradiance, i.e., the accelerated radiative decay of the atomic ensemble. On the energy scale this corresponds to an enhanced decay width, i.e., a broadening of the resonance line. Another result of the collective coupling is a shift of the transition energy, the collective Lamb shift (CLS). In a similar fashion as the atomic Lamb shift is a self-energy correction of the transition energy, the collective Lamb shift is a self-energy correction to the transition energy of the ensemble. Both effects, superradiance and collective Lamb shift, depend critically on the spatial arrangment of the ensemble of emitting atoms. This plays an important role in light-harvesting systems, were superradiant entanglement of the atoms is crucial for fast transfer of energy into the system.
Mössbauer nuclei are (almost) ideal two-level systems to study these phenomena, as will be illustrated in the following.
Observation of the Collective Lamb Shift in Single-Photon Superradiance
The development of quantum electrodynamics is very closely related to the discovery and explanation of the Lamb shift of atomic energy levels [1, 2]. The Lamb shift is a tiny energy shift of bound atomic states due to the interaction of the atomic charges with the vacuum fluctuations of the electromagnetic field. The main contribution to the Lamb shift results from the emission and reabsorption of virtual photons within the same atom.
An additional contribution to the Lamb shift emerges if many identical atoms are interacting collectively with a resonant radiation field. In this case a virtual photon that is emitted by one atom may be reabsorbed by another atom within the ensemble. The resulting collective Lamb shift was already calculated more than 35 years ago [3] and just recently revisited in the connection with single-photon Dicke superradiance [4-7]. Since the early theoretical work only one measurement of an excited-state collective Lamb shift has been reported for a multiphoton excitation scheme in a noble gas [8]. The experimental assessment of the collective Lamb shift for groundstate transitions, particularly in solid-state samples, has remained elusive.
Recently, we could measure the collective Lamb shift for an ensemble of Mössbauer nuclei that was resonantly excited by synchrotron radiation x-rays [9]. In order to prepare a state with a well-defined collective Lamb shift, the nuclei were embedded in the center of a planar waveguide into which the x-rays were evanescently coupled. This ensured the nuclei being prepared in an entangled, purely superradiant state, constituting the key ingredient for measuring the collective Lamb shift.
These results demonstrate that planar cavities can be employed in a rather simple fashion to create superradiant states of excited atoms in general. We anticipate that this approach will stimultate further studies of cooperative phenomena in optics, reaching far out into the field of quantum optics with x-rays.
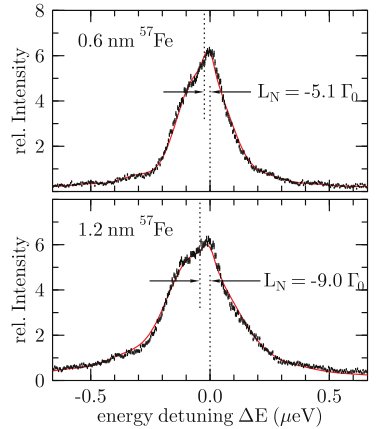
The measured energy spectra exhibit a redshift that corresponds to the collective Lamb shift, in excellent agreement with theory.
| References |
|
[1] W. E. Lamb, Jr., and R. C. Retherford, Phys. Rev. 72, 241 (1947). |






